KLIPPEL ANALYZER SYSTEM DC Displacement with 3D-Distortion Measurement (DIS)

Driver Name:	SB 12
Driver Comment:
Measurement:	DIS Fundamental, DC
Measurement Comment:	Used for analyzing DC offset and its causes
Date:	07/21/07
Time:	19:45:59

---

Comments

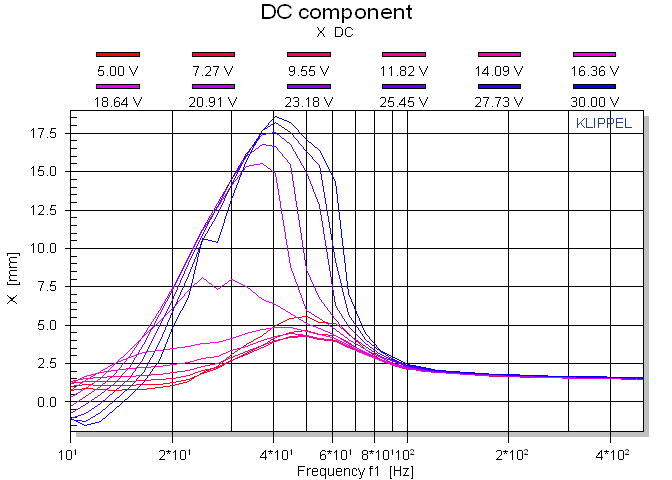
Substantial DC offset or rectification (17.5+mm) above resonance. This is probably caused by a combination of the coil rest position, and extremely high compliance (Very low Fo). See additional documents for more details and solutions.

Overview

The dc-component in the displacement will be generated dynamically if nonlinearities (Bl(x)-product, compliance Cms(x) and Le(x)) have an asymmetrical characteristic. At the resonance frequency the asymmetry of the stiffness is usually the dominant source for DC. Above the resonance frequency the force factor (Bl(x)-product) contributes more and more. If the motor nonlinearity is large and the compliance is low the system becomes instable f=2fs pushing the coil literally out to the gap. A generated dc-component will destroy the optimal working point. For example a dc-component generated by asymmetric stiffness may shift the voice coil position producing substantial Bl-distortion at higher frequencies eventually.

Thus ensuring a small DC component is a essential requisition for ensuring stable performance and low distortion in the large signal domain.

Physics of generating a DC-displacement

Causes

There are two mechanisms that generate a DC component in the displacement.

1. Any asymmetry in the nonlinear characteristic of the electrical and mechanical parameters (partly) rectifies the AC signal and produces a DC component as well as second-order and higher-order distortion. The DC component has a much higher amplitude than any other harmonic and intermodulation component if the transducer is excited by a complex signal. The reason for this is that the DC component is accumulated by rectifying any fundamental component whereas the other distortion components are distributed over the whole frequency band.

2. An electro-dynamical motor which has a perfect symmetrical Bl(x) characteristic may become unstable if the stiffness of the suspension is very low and the driver is operated above the resonance frequency. Any small disturbance at the rest position will ignite the generation of a DC component and the coil slides down the Bl(x) slope until the suspension has produced a restoring force large enough to stop this process.

Orientation

The sign of the DC displacement determines the direction of the voice coil shift. In this application note positive displacements x denote shifts that move the coil away from the backplate (coil out).

Direction

The direction of the DC displacement depends on the shape (extrema, asymmetry) of the transducer nonlinearities such as Cms(x), Bl(x) and Le(x) and on the frequency of the excitation tone. The DC displacement caused by an asymmetric compliance moves the coil always towards the direction of the stiffness minimum. An asymmetric inductance causes a DC component that moves the coil towards higher inductance values – similar to the attraction force in a electromagnet. The DC component produced by the force factor Bl(x) depends on the frequency of the fundamental component. For frequencies below the resonance frequency the coil is moved towards the maximum of the Bl(x) curve. This means that the coil is self-centring which is a nice feature. unfortunately, the same motor will push the coil away from the Bl(x) maximum for any frequency above the resonance.

Crossing Point

Some loudspeakers produce both a positive and negative DC displacement depending on the frequency of the excitation tone. At the point where positive displacement changes to negative and vice versa (crossing point) all the DC forces produced by the different rectification processes cancel out each other. This point is quite reproducible and almost independent of the magnitude of the DC component.

Influence of the suspension creep

The DC displacement of real world transducers varies with ongoing operation. After starting to operate the transducer an initial DC component is generated. The magnitude of the DC displacement depends among others on the stiffness of the suspension at very low frequencies (f ˜ 0 Hz). However, the stiffness of the suspension of real transducers is frequency dependent. Usually, the suspension is much stiffer at the resonance frequency than at very low frequencies (corresponding to very slow cone movements). Any displacement of the suspension will cause changes in the geometry of the fibres of

the rubber and fabric and the relocation time has a time constant in the order of magnitude of 1s. The loss of stiffness at lower frequencies is described by the creep factor which can be measured with LPM software module of the Klippel Analyzer System. The DC force will produce a variable DC displacement depending on the creep factor and the measurement time.

Effects of dominant nonlinearities
NONLINEARITY	FREQUENCY OF THE EXCITATION TONE
	f < fs	f = fs	f>fs	f>>fs
Bl(x) Motor	moves to Bl(x) maximum	no DC component	moves coil away Bl(x) maximum (unstable)	negligible
Cms(x) Suspension	moves coil to stiffness minimum	moves coil to stiffness minimum	negligible	negligible
Le(x) Reluctance force	moves coil to Le(x) maximum	negligible	moves coil to Le(x) maximum	moves coil to Le(x) maximum

Fundamental (mm / Rms)

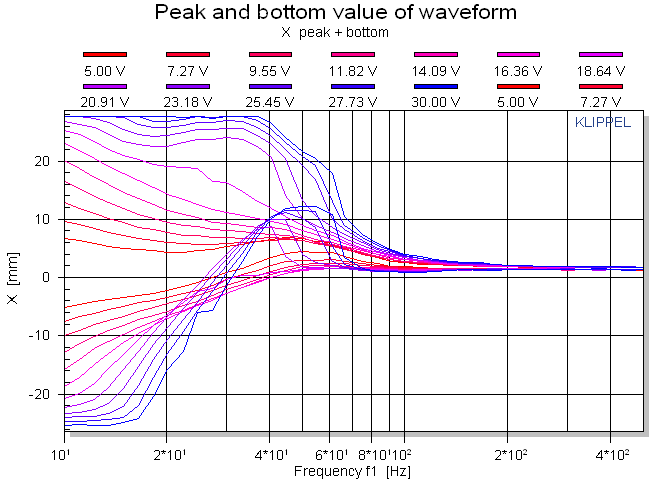
DC Component (mm Peak)

The figure below shows the dc component in voice coil displacement for varied voltage U and frequency f. of the sinusoidal excitation tone.